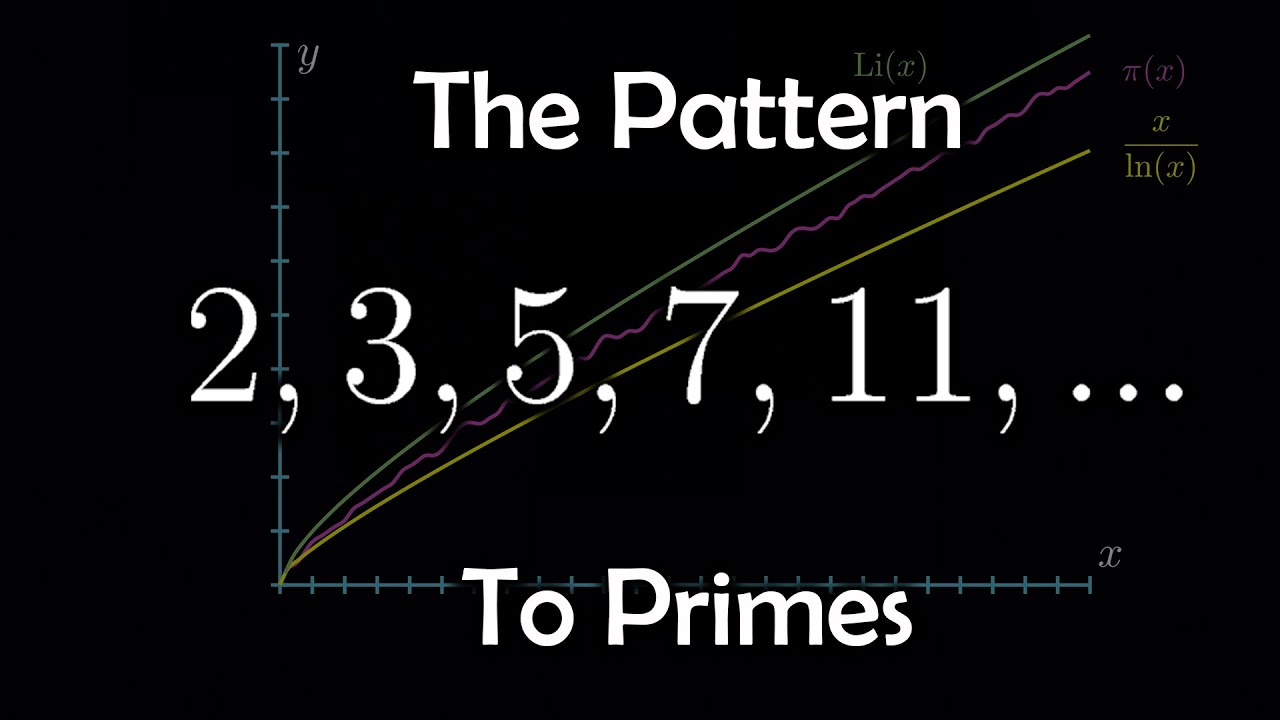
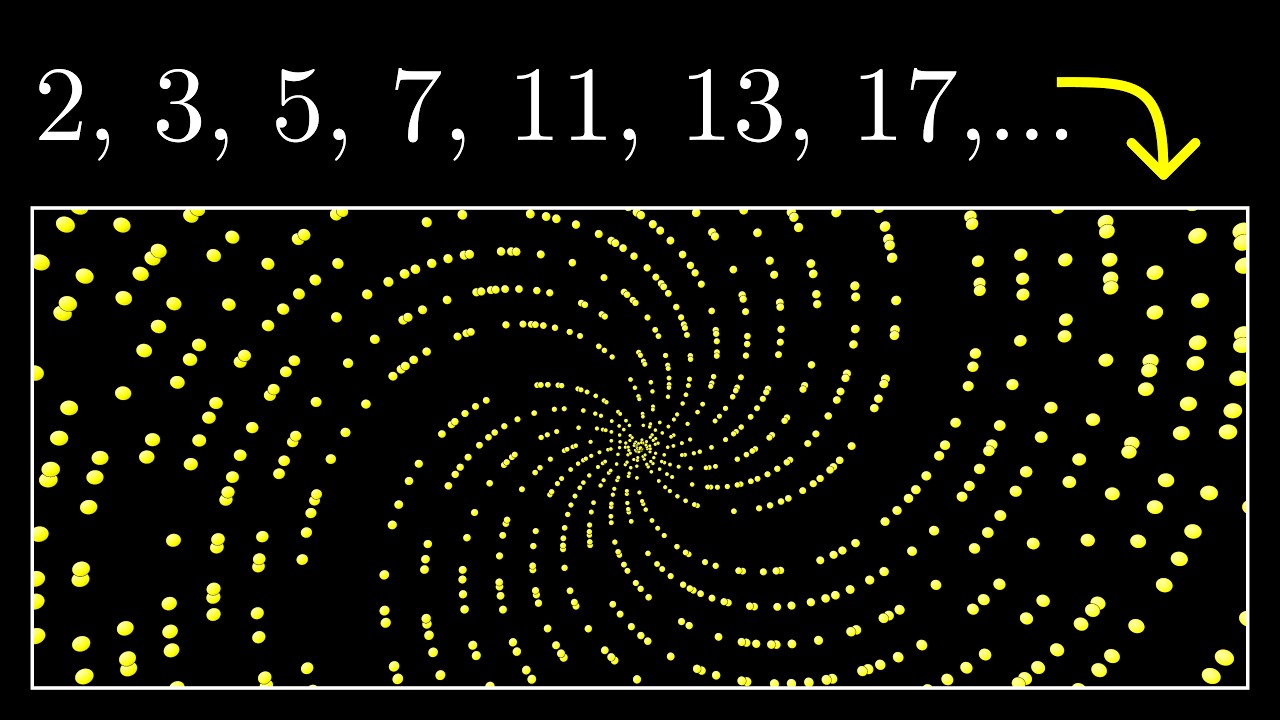
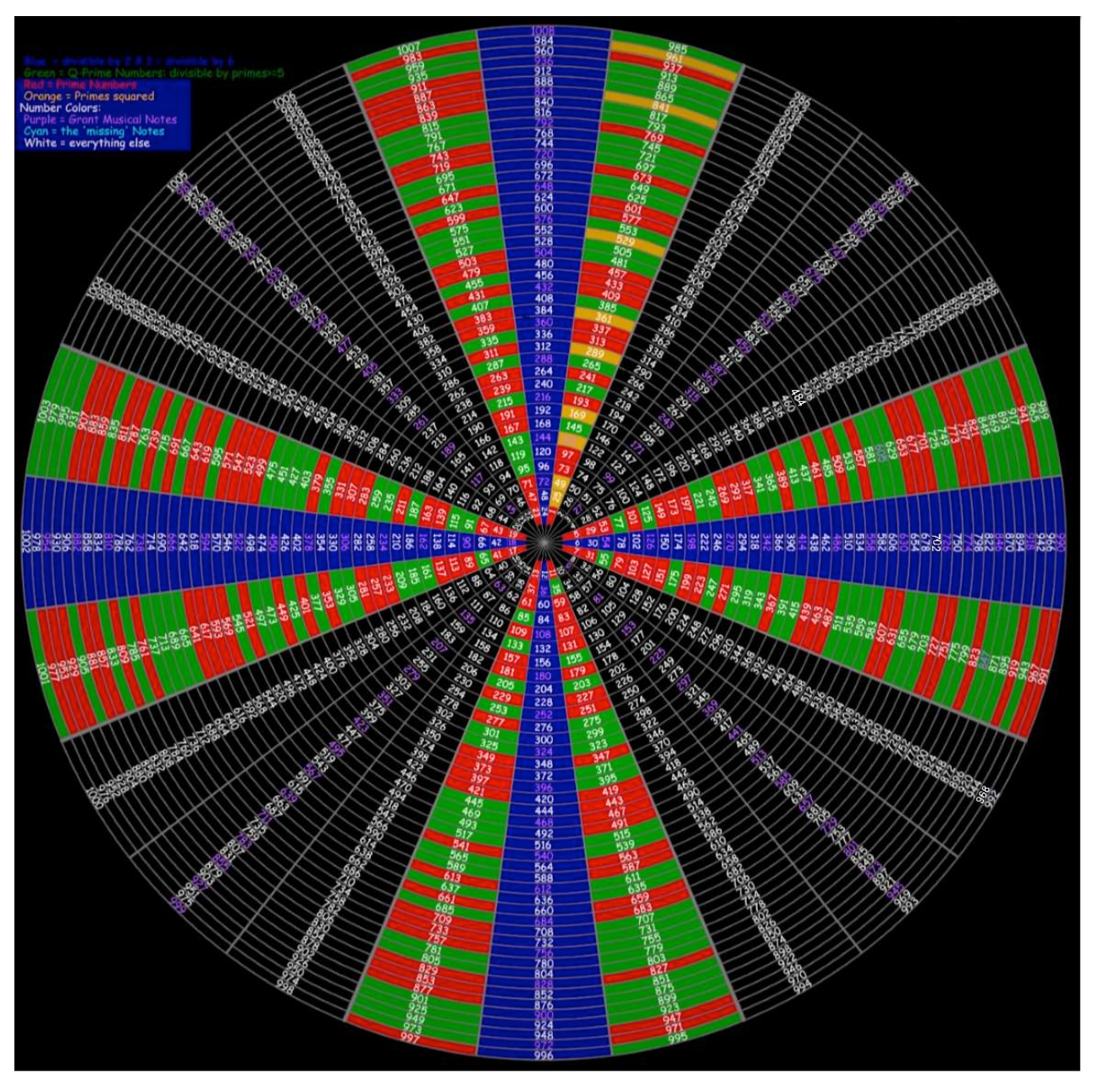
Is There A Pattern To Prime Numbers
Is There A Pattern To Prime Numbers - I think the relevant search term is andrica's conjecture. The find suggests number theorists need to be a little more careful when exploring the vast. The other question you ask, whether anyone has done the calculations you have done, i'm sure the answer is yes. Web patterns with prime numbers. For example, is it possible to describe all prime numbers by a single formula? Web two mathematicians have found a strange pattern in prime numbers—showing that the numbers are not distributed as randomly as theorists often assume. They prefer not to mimic the final digit of the preceding prime, mathematicians have discovered. As a result, many interesting facts about prime numbers have been discovered. This probability becomes $\frac{10}{4}\frac{1}{ln(n)}$ (assuming the classes are random). Web the probability that a random number $n$ is prime can be evaluated as $1/ln(n)$ (not as a constant $p$) by the prime counting function. The find suggests number theorists need to be a little more careful when exploring the vast. Quasicrystals produce scatter patterns that resemble the distribution of prime numbers. Are there any patterns in the appearance of prime numbers? Web prime numbers, divisible only by 1 and themselves, hate to repeat themselves. Many mathematicians from ancient times to the present have studied prime numbers. Web two mathematicians have found a strange pattern in prime numbers — showing that the numbers are not distributed as randomly as theorists often assume. Web two mathematicians have found a strange pattern in prime numbers—showing that the numbers are not distributed as randomly as theorists often assume. If we know that the number ends in $1, 3, 7, 9$; Web mathematicians are stunned by the discovery that prime numbers are pickier than previously thought. This probability becomes $\frac{10}{4}\frac{1}{ln(n)}$ (assuming the classes are random). The other question you ask, whether anyone has done the calculations you have done, i'm sure the answer is yes. The find suggests number theorists need to be a little more careful when exploring the vast. Web prime numbers, divisible only by 1 and themselves, hate to repeat themselves. Quasicrystals produce scatter patterns that resemble the distribution of prime numbers.. The find suggests number theorists need to be a little more careful when exploring the vast. Web prime numbers, divisible only by 1 and themselves, hate to repeat themselves. Web mathematicians are stunned by the discovery that prime numbers are pickier than previously thought. Web the probability that a random number $n$ is prime can be evaluated as $1/ln(n)$ (not. The find suggests number theorists need to be a little more careful when exploring the vast. Web patterns with prime numbers. The other question you ask, whether anyone has done the calculations you have done, i'm sure the answer is yes. Web two mathematicians have found a strange pattern in prime numbers — showing that the numbers are not distributed. The other question you ask, whether anyone has done the calculations you have done, i'm sure the answer is yes. I think the relevant search term is andrica's conjecture. This probability becomes $\frac{10}{4}\frac{1}{ln(n)}$ (assuming the classes are random). Web patterns with prime numbers. Many mathematicians from ancient times to the present have studied prime numbers. I think the relevant search term is andrica's conjecture. If we know that the number ends in $1, 3, 7, 9$; They prefer not to mimic the final digit of the preceding prime, mathematicians have discovered. Web two mathematicians have found a strange pattern in prime numbers—showing that the numbers are not distributed as randomly as theorists often assume. Web. They prefer not to mimic the final digit of the preceding prime, mathematicians have discovered. Quasicrystals produce scatter patterns that resemble the distribution of prime numbers. Web patterns with prime numbers. This probability becomes $\frac{10}{4}\frac{1}{ln(n)}$ (assuming the classes are random). Web two mathematicians have found a strange pattern in prime numbers — showing that the numbers are not distributed as. Quasicrystals produce scatter patterns that resemble the distribution of prime numbers. Web mathematicians are stunned by the discovery that prime numbers are pickier than previously thought. As a result, many interesting facts about prime numbers have been discovered. Web patterns with prime numbers. Many mathematicians from ancient times to the present have studied prime numbers. Are there any patterns in the appearance of prime numbers? Web the probability that a random number $n$ is prime can be evaluated as $1/ln(n)$ (not as a constant $p$) by the prime counting function. For example, is it possible to describe all prime numbers by a single formula? This probability becomes $\frac{10}{4}\frac{1}{ln(n)}$ (assuming the classes are random). As a. I think the relevant search term is andrica's conjecture. For example, is it possible to describe all prime numbers by a single formula? The find suggests number theorists need to be a little more careful when exploring the vast. Web patterns with prime numbers. They prefer not to mimic the final digit of the preceding prime, mathematicians have discovered. Quasicrystals produce scatter patterns that resemble the distribution of prime numbers. Web the probability that a random number $n$ is prime can be evaluated as $1/ln(n)$ (not as a constant $p$) by the prime counting function. For example, is it possible to describe all prime numbers by a single formula? Web now, however, kannan soundararajan and robert lemke oliver of. If we know that the number ends in $1, 3, 7, 9$; Web the probability that a random number $n$ is prime can be evaluated as $1/ln(n)$ (not as a constant $p$) by the prime counting function. Quasicrystals produce scatter patterns that resemble the distribution of prime numbers. Web mathematicians are stunned by the discovery that prime numbers are pickier than previously thought. Web two mathematicians have found a strange pattern in prime numbers — showing that the numbers are not distributed as randomly as theorists often assume. For example, is it possible to describe all prime numbers by a single formula? The find suggests number theorists need to be a little more careful when exploring the vast. Web prime numbers, divisible only by 1 and themselves, hate to repeat themselves. Web two mathematicians have found a strange pattern in prime numbers—showing that the numbers are not distributed as randomly as theorists often assume. Many mathematicians from ancient times to the present have studied prime numbers. They prefer not to mimic the final digit of the preceding prime, mathematicians have discovered. Web the results, published in three papers (1, 2, 3) show that this was indeed the case: This probability becomes $\frac{10}{4}\frac{1}{ln(n)}$ (assuming the classes are random). The other question you ask, whether anyone has done the calculations you have done, i'm sure the answer is yes. Web now, however, kannan soundararajan and robert lemke oliver of stanford university in the us have discovered that when it comes to the last digit of prime numbers, there is a kind of pattern.Prime Numbers Definition, Examples, Properties, Gaps, Patterns
[Math] Explanation of a regular pattern only occuring for prime numbers
Plotting Prime Numbers Jake Tae
Prime number patterns Prime numbers, Number theory, Geometry
Prime Number Patterning! The Teacher Studio Learning, Thinking, Creating
The Pattern to Prime Numbers? YouTube
A Pattern in Prime Numbers ? YouTube
Prime Numbers Definition, Prime Numbers 1 to 100, Examples
Why do prime numbers make these spirals? Dirichlet’s theorem and pi
Prime Number Pattern Discovery PUBLISHED
Are There Any Patterns In The Appearance Of Prime Numbers?
As A Result, Many Interesting Facts About Prime Numbers Have Been Discovered.
Web Patterns With Prime Numbers.
I Think The Relevant Search Term Is Andrica's Conjecture.
Related Post:
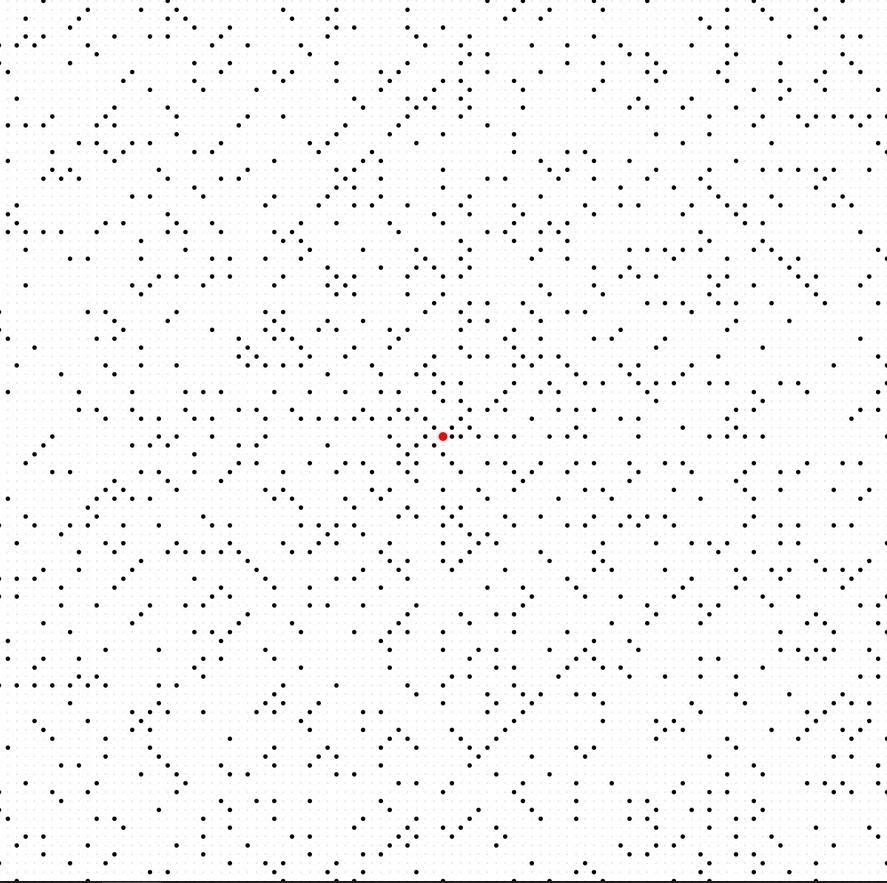
![[Math] Explanation of a regular pattern only occuring for prime numbers](https://i.stack.imgur.com/N9loW.png)